Quantum Mechanics 1 | Week 5
Session: JAN-APR 2024
Course name: Quantum Mechanics I
Course Link: Click Here
For answers or latest updates join our telegram channel: Click here to join
These are Quantum Mechanics 1 Week 5 Assignment Nptel Answers
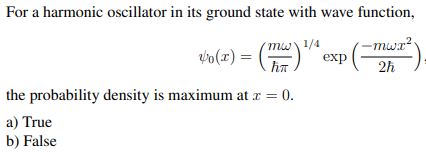
Q1. Consider the states 4) = 3101) 7i|02) and (x) = -1) + 2i|02), where 1) and (2) are orthonormal. The states satisfy the triangle inequality.
a) True
b) False
Answer: a) True
Q2. Consider two operators A and B which are Hermitian and commute. Then the operator (A+B)/√(A² + B²) is unitary.
a) True
b) False
Answer: a) True
Q3. Consider a one-dimensional particle with the wavefunction
(x,t) = sin(x/a) exp(-iwt)
which is confined within the region 0 ≤ x ≤ a. The probabilty of finding the particle in the interval a/4 < x <3a/4 is __________. (Write upto two decimal places.)
Answer: 0.82
For answers or latest updates join our telegram channel: Click here to join
These are Quantum Mechanics 1 Week 5 Assignment Nptel Answers
Q4. A system is intially in the state = [√21) + √302) + 3) + 4)]/√7, where on) are the eigenstates of the system’s Hamiltonian such that H) = n2Eon). If energy E₂ is measured, its value and the probability will be respectively,
a) 480, 3/7
b) 480, √3/√7
c) E0, 3/7
d) Eo, √6/√7
(Here & is the ground state energy of the system.)
Answer: a) 480, 3/7
Q5. If (l, m [Lz, A]|l, m) = iħ for any observable A, then the expectation value of 2 p is
a) -i
b) 1
c) 0
d) -1
Answer: b) 1
Q6. Consider the one dimensional motion for a free particle and three operators, the Hamiltonian H, the momentum operator p and the parity operator P. Which of the following are commuting?
a) [H, p2]
b) [HP]
c) [P, p²]
d) [P.p
Answer: a), b), c)
For answers or latest updates join our telegram channel: Click here to join
These are Quantum Mechanics 1 Week 5 Assignment Nptel Answers
Q7. The initial wavefunction of a particle in the harmonic oscillator potential (mw²²) can be written as
(х, 0) = A[340(x) + 441(x)],
where A is the normalization constant, 40 and 1 represent the ground state and first excited states of the harmonic oscillator respectively and are orthogonal. Then (x, t)|2 can be written as b + c + d4041 cos(wt)], where
i) a = _______(Answer should be an integer)
ii) b = _______(Answer should be an integer)
iii) c = _______(Answer should be an integer)
iv) d = _______(Answer should be an integer)
Answer: 25,9,16,24
Q8. The Hamiltonian operator for a two state system is given by
H = a[2|1) (131) (22) (1|]
where a is a number with dimensions of energy. The corresponding eigenvalues and eigenvectors will be
a) a (1±√2); ((-√21) + (2)), (√21) + (2)))
b) ±a; ((11) +12)), (11)-(2)))
с) а, -3а; ((31) (31) + 2)), (11) + (2))) (11)+|
d) -a, 3а; ((-31) + (2)), (11) + (2)))
Answer: d) -a, 3а; ((-31) + (2)), (11) + (2)))
These are Quantum Mechanics 1 Week 5 Assignment Nptel Answers
Q9. Suppose a particle is in state, P)=(31) 2i 2) + √7/3)), where {11), (2), (3)} form an orthonormal basis. For an observable B = a1)(1 + b/3) (3), the probability of measuring bin such a state is
(a) 0
(b) 3/10
(c) 8/25
(d) 7/20
Answer: (d) 7/20
These are Quantum Mechanics 1 Week 5 Assignment Nptel Answers
Q10. In a system described by three orthonormal basis (1), (2), (3), the operator form of an observable is A = 7|1) (1| +92) (25/3) (3). The possible measurable values of observable  will be
a) -7, -9, 5
b) 7, 9, -5
c) 1/7, 1/9, -1/5
d) -1/7, -1/9, 1/5
Answer: b) 7, 9, -5
For answers or latest updates join our telegram channel: Click here to join
These are Quantum Mechanics 1 Week 5 Assignment Nptel Answers
More Weeks of Quantum Mechanics I: Click here
More Nptel Courses: https://progiez.com/nptel-assignment-answers
| The content uploaded on this website is for reference purposes only. Please do it yourself first. |